How Does Light Get Pulled Into a Black Hole
A Black Hole is a Waterfall of Space
Definition of a black hole
You may well have heard the popular definition of a black hole. A black hole is an object whose gravity is so strong that not even light can escape. The definition is correct, but it does not give a good picture of why light cannot escape.
I prefer a different definition. A black hole is a place where space is falling faster than light.
Ren and Stimpy


One of the most popular "Ren & Stimpy" episodes
is "Black Hole", in which Ren & Stimpy get pulled into a black hole.
Inside the black hole, Ren & Stimpy find themselves on a strange planet where bizarre things happen. They morph into weird shapes. Body parts detach and reassemble.
The episode is a delightful artistic interpretation of the idea that black holes are places where space and time get fantastically warped. The idea is correct, but it does not give much to hang your brain on if you want to understand what really happens inside black holes.
A misleading picture

In 1784, John Michell1 proposed, in the context of Newton's corpuscular theory of light, that if the escape velocity from a mass exceeded the speed of light, then the mass would be invisible. In his picture, light emitted from the object would go up, curve around, and come back down.
Michell's picture is enticing, but it is different from what general relativity predicts. General relativity predicts that any light emitted within the horizon is dragged inward, even if the light is pointed directly outward. The picture at left, which shows light going up, turning around, and falling back down, is misleading.
Waterfall of space


A more insightful way to conceptualize how a black hole works is to picture space as flowing like a waterfall into the black hole. At left is a movie of Boulder Falls that I photographed. Here's the movie with sound.
Imagine light rays, photons, as fishes swimming fiercely in the current. Outside the horizon, space is falling into the black hole at less than the speed of light (or the speed of fishes), and photon-fishes swimming upstream can make way against the flow. At the horizon, space is falling into the black hole at the speed of light. At the horizon a photon-fish swimming directly upstream will just stay there, swimming like crazy, but not going anywhere, the inward flow of space exactly canceling the fish's motion. Inside the horizon, the space waterfall falls faster than the speed of light, carrying everything with it. However hard it tries to swim upstream, the photon-fish inside the horizon is carried by the flow of space inevitably inward to its ultimate fate.
In the image at right, the (happy) fish upstream can make way against the current, but the (sad) fish downstream is swept to the bottom of the waterfall. This picture was drawn by my daughter Wild, and provided the cover image for the June 2008 issue of the American Journal of Physics4.
Doesn't relativity say that nothing can go faster than light? It is true that nothing can travel through space faster than light. However, in general relativity, space itself can do whatever it likes.
The idea of space moving is one that you may have met before in cosmology (the study of the Universe at large), in the notion that the Universe expands.
The Schwarzschild waterfall
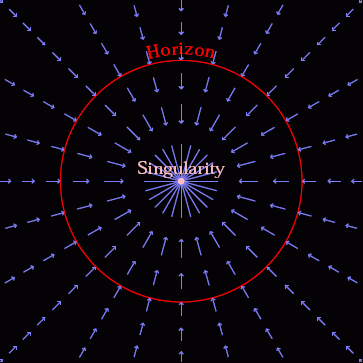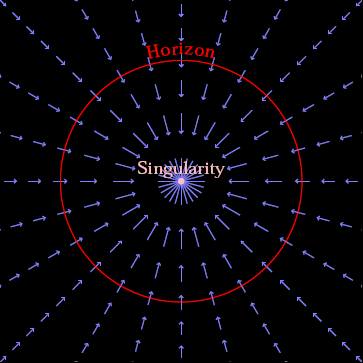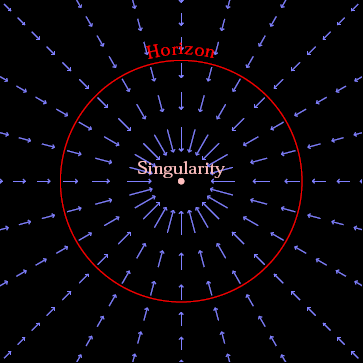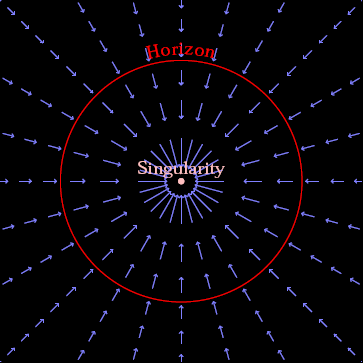
The picture of spacing falling into a black hole has a sound mathematical basis, first discovered in 1921 by the Nobel prize-winner Alvar Gullstrand2, and independently by the French mathematician and politician Paul Painlevé3, who was Prime Minister of France in 1917 and then again in 1925.
It is not necessary to understand the mathematics, but I do want to emphasize that, because the concept of space falling into a black hole is mathematically correct4, inferences drawn from that concept are correct.
The Gullstrand-Painlevé metric is
\( d s^2 = - \, d t_{\rm ff}^2 + ( d r - v \, d t_{\rm ff} )^2 + r^2 ( d \theta^2 + \sin^2\theta \, d \phi^2 ) \)
which is just the Schwarzschild metric expressed in a different coordinate system. The free-fall time \(t_{\rm ff}\) is the proper time experienced by observers who free-fall radially from zero velocity at infinity. The velocity \(v\) in the Gullstrand-Painlevé metric equals the Newtonian escape velocity from a spherical mass \(M\)
\( \displaystyle v = - \sqrt{2 G M \over r} \)
with a minus sign because space is falling inward, to smaller radius.
Physically, the Gullstrand-Painlevé metric describes space falling into the Schwarzschild black hole at the Newtonian escape velocity. Outside the horizon, the infall velocity is less than the speed of light. At the horizon, the velocity equals the speed of light. And inside the horizon, the velocity exceeds the speed of light. Technically, the Gullstrand-Painlevé metric encodes not only a metric, but also a complete orthonormal tetrad, a set of four locally inertial axes at each point of the spacetime. The Gullstrand-Painlevé tetrad free-falls through the coordinates at the Newtonian escape velocity.
It is an interesting historical fact that the mathematics of black holes was understood long before the physics. Einstein himself misunderstood how black holes work. He thought that the Schwarzschild geometry had a singularity at its horizon, and that the regions inside and outside the horizon constituted two separate spacetimes. I think that even today research into general relativity is too often dominated by abstract mathematical thinking at the expense of conceptual understanding.
The Reissner-Nordström waterfall

The waterfall model works equally well for a charged black hole, the Reissner-Nordström geometry. However, whereas in the Schwarzschild geometry the waterfall falls at ever increasing velocity all the way to a central singularity, in the Reissner-Nordström geometry the waterfall slows down, thanks to the gravitational repulsion produced by the tension, or negative pressure, of the electric field.
The Reissner-Nordström waterfall is described by exactly the same Gullstrand-Painlevé2,3 metric as the Schwarzschild metric, but the mass \(M\) in the escape velocity is replaced by the mass \(M(r)\) interior to radius \(r\):
\( \displaystyle v = - \sqrt{2 G M(r) \over r} \) .
The interior mass \(M(r)\) equals the mass \(M\) as seen at infinity, minus the mass-energy \(Q^2 / ( 2 r )\) in the electric field
\( \displaystyle M(r) = M - {Q^2 \over 2 r} \) .
The electromagnetic mass \(Q^2 / ( 2 r )\) is the mass outside \(r\) associated with the energy density \(E^2 / (8\pi)\) of the electric field \(E = Q / r^2\) surrounding a charge \(Q\).
The infall velocity \(v\) of space passes the speed of light c at the outer horizon \(r_+ = M + (M^2 - Q^2)^{1/2}\), but slows back down to less than the speed of light at the inner horizon \(r_- = M - (M^2 - Q^2)^{1/2}\). The velocity slows all the way to zero at the turnaround point \(r_0 = Q^2 / (2 M)\) inside the inner horizon. At this point space turns around and accelerates back out, hitting the speed of light once more at the inner horizon \(r_-\). Space now enters a white hole, where space is moving outward faster than light. The diagram at left seems to show the white hole in the same place as the black hole, but actually, as is apparent in the Penrose diagram, the white hole and black hole are distinct regions of spacetime. As space falls outward in the white hole, the gravitational repulsion produced by the negative pressure of the electric field weakens relative to the gravitational attraction produced by the mass. The outfalling space slows back down to the speed of light at the outer horizon \(r_+\) of the white hole. Space then emerges into a new region of spacetime, a new universe perhaps.
Does all this sound bizarre? Yes it is. Sadly, the whole picture of space turning around and shooting back out through a white hole into another spacetime does not happen in reality. Although the Reissner-Nordström geometry is an exact mathematical solution of Einstein's equations, in reality the geometry is subject to a violent instability at the inner horizon.
Wormhole waterfall

When did you last see a waterfall that cascaded down one side of a ravine and leapt up the other?
Well, that doesn't happen in reality, and it doesn't happen in black holes either.
In a waterfall, water bouncing off the bottom of the ravine collides with water streaming down the falls, producing a turbulent chaos of water at the bottom of the waterfall.
Something not greatly different happens inside a charged or rotating black hole. Since space has no substance (according to relativity), as long as the black hole is empty, then there is no problem with space going in two different directions at once. But the moment any actual stuff falls into the black hole, as it surely does in reality, then stuff trying to fly back out collides with stuff still falling in. As at the bottom of a waterfall, the result is energetic chaos deep inside the black hole.
A mathematician might construct a solution in which a waterfall fell down one side of a ravine, turned smoothly around, and fell up the other side, conserving energy and momentum, and generating no entropy. The mathematician might argue that indeed that is the simplest mathematical solution for a waterfall. But in reality such a waterfall never happens.
The Kerr waterfall

The geometry of a rotating black hole is described by the Kerr metric, discovered by the New Zealander Roy Kerr in 19635. The Kerr geometry shares with the Reissner-Nordström geometry the property of having an inner horizon, which connects to a white hole, which connects to a new universe.
The centrifugal force causes the horizon of the black hole to bulge out into an ellipse. The same centrifugal force opens the singularity into a ring. The outer horizon and inner horizon form confocal ellipsoids, with the ring singularity at the focus of the ellipsoid.
The rotating black hole drags space around with it. Outside the horizon of the black hole is a region called the ergosphere, where space is dragged around so fast that nothing can remain at rest there. A former student once called the ergosphere the place where little children come from, because nothing can remain at rest there.
The Kerr antiverse

If you pass through the disk bounded by the ring singularity of the Kerr black hole, then you enter a different region of space, the antiverse. The antiverse continues the Kerr geometry to negative radius (in ellipsoidal coordinates). An observer in the antiverse perceives the Kerr geometry to be a rotating black hole of negative mass.
Winding around the ring singularity is a toroidal region, which I call the sisytube, containing closed timelike curves (CTCs)6. An observer who circulates retrograde around the torus can find themself joining to their own past. Like Sisyphus, they are doomed to repeat the same loop of time for ever.
Sadly, from its inner horizon inward, the Kerr geometry is not a realistic model of an astronomical black hole, because the Kerr geometry is subject to the inflationary instability at its inner horizon. Click on Realistic to learn more about the inflationary instability.
References
- John Michell (1784) "On the Means of Discovering the Distance, Magnitude, Etc., of the Fixed Stars, in Consequence of the Diminution of their Light, in case such a Diminution Should Be Found to Take Place in Any of the Them, and Such Other Data Should be Procured from Observations, as Would Be Futher Necessary for That Purpose" Phil. Trans. Roy. Soc. London 74 35
- Allvar Gullstrand (1922) "Allgemeine Lösung des statischen Einkörperproblems in der Einsteinschen Gravitationstheorie" (translated by the now defunct web tool babelfish as "General solution of the static body problem in Einstein's Gravitation Theory") Arkiv. Mat. Astron. Fys. 16(8) 1-15
- Paul Painlevé (1921) "La mécanique classique et la théorie de la relativité" C. R. Acad. Sci. (Paris) 173 677-680
- Andrew J. S. Hamiton & Jason P. Lisle (2008) "The river model of black holes" Am. J. Phys. 76 519-532, gr-qc/0411060
- Roy P. Kerr (1963) "Gravitational field of a spinning mass as an example of algebraically special metrics" Phys. Rev. Lett. 11 237--238
- Brandon Carter (1968) "Global structure of the Kerr family of gravitational fields" Phys. Rev. 174 1559-1571
How Does Light Get Pulled Into a Black Hole
Source: https://jila.colorado.edu/~ajsh/insidebh/waterfall.html